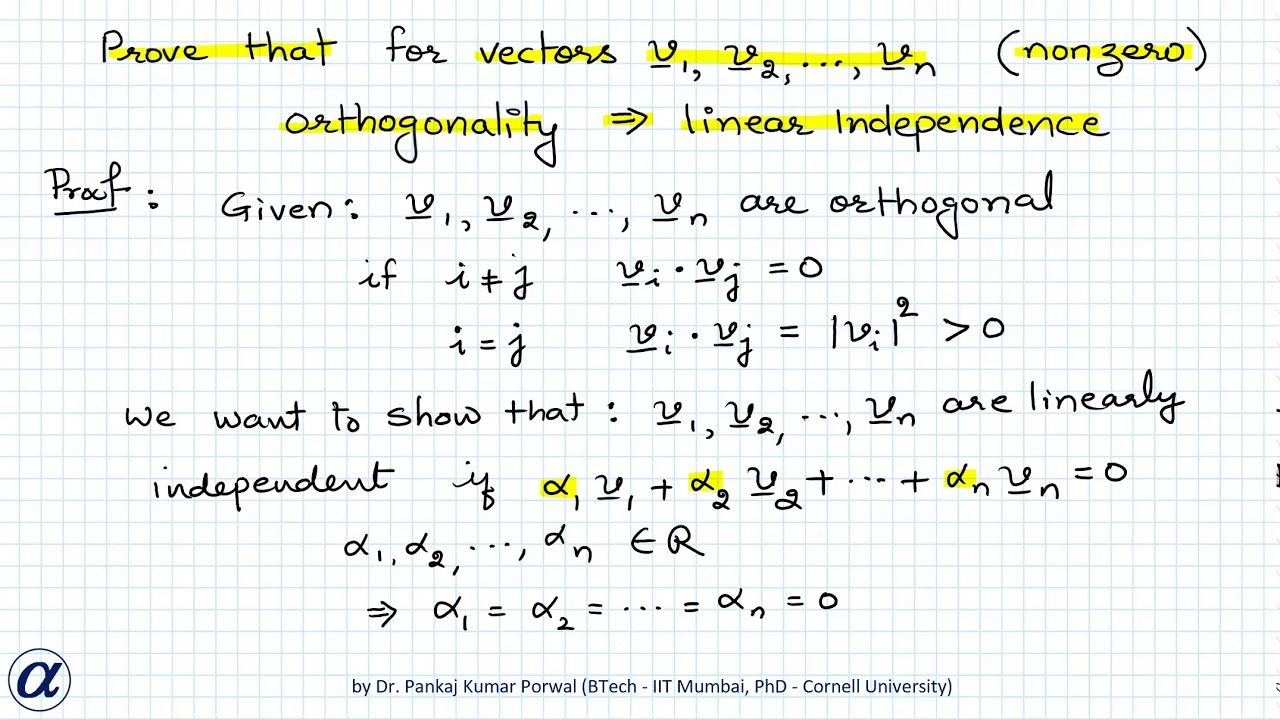
Orthogonality implies Linear Independence ( Orthogonal implies Linearly Independent )

Prove that Orthogonality implies Linear Independence


Linear Independence
A collection of vectors is called linearly independent if cannot write any vector in the collection as linear combination of remaining vector. Linear independence is the properties of the collection. We cannot pick few vectors from the collection and check for linear independence of the collection.

Orthogonality
A collection of vectors is orthogonal if each pair of vectors in orthogonal, i.e., perpendicular to each other. In other words dot product of each pair of vectors in the collection is zero.

Orthonormality
A collection of vectors is orthonormal if
(i) each pair of vectors is orthogonal
(ii) each vector in the collection has unit magnitude.

Orthonormality implies orthogonality.
Orthonormality implies linear independence.
Orthogonality implies linear independence.

What is meaning of linear independence of vectors ?
What is meaning of orthogonality of vectors ?
What is the meaning of orthonormality of vectors ?
Does linear independence imply orthogonality ?
Does linear independence imply orthonormality ?
Does orthonormality imply orthogonality ?
Does orthonormality imply orthogonality ?
Does orthogonality imply linear independence ?




This video is uploaded by
Alpha Academy, Udaipur

Minakshi Porwal (9460189461)

Vector,scaler,Vector space,Span,Linear combination,Closed,Under,Addition,Scaler multiplication,Basis,Linearly independent,Linear independence,coordinates,Dimension,Orthogonal,Perpendicular,Normal,Orthonormal,GATE,IIT,concept,understand,understanding,trick,short cut,short trick,short,quick,fast,easy,simple,solution,answer,basic,fundamental,Rank,Nullity,Range Space,Null Space,